MARIANO TOMATIS

Curse of the crystal skulls and other vanishing area puzzles

As in Arthur C. Clarke’s “The Nine Billion Names of God” (1953), mathematics can provide a shortcut to the Day of Judgement. But... is it worthwhile?

The idea came to my mind during a congress, when I gave a talk just before Gianni Sarcone, who showed this vanishing area puzzle:

I needed to design a puzzle for my book about the end of the world in 2012 and I asked Gianni to reshape the picture of 7/8 eggs to support the narrative of 12/13 crystal skulls. The result was published in 2012: The end-of-the-world game (1):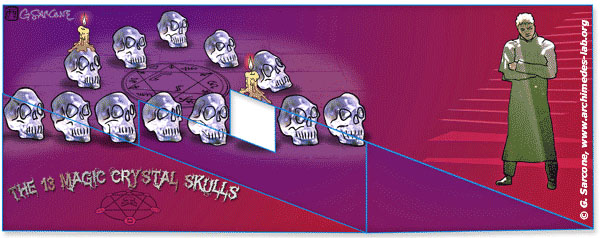

First vanishing area puzzles in print by Sebastiano Serlio

The first example of vanishing area puzzles was reported in the book Libro d’Architettura Primo by Sebastiano Serlio (1475-1554), an Italian architect of the Renaissance. (2):

Sometimes strange accidents happen to the architect: in situations like the one here described, the methods of mathematicians will help. He has a single panel 10 feet × 3 feet and he needs to convert it into a 7 feet × 4 feet panel. Cutting it vertically, its height will double and will reach 6 feet but he needs 7. At the same time, cutting a lateral slice of 3 feet, it will not satisfy the need because the remaining part will have a size of 7 feet × 3 feet. In order to get the result, he has to follow these instructions. The panel, 10 feet × 3 feet, has four vertices A, B, C, D: cut it on its diagonal CB and slide the upper part towards B until the distance between the point A and its projection F will be 4 feet. The same will happen with the distance ED, and the distance AE will be 7 feet, so that the new panel AEFD will be 7 feet × 4 feet. Two additional triangles allow to create a small door: CFC and EBG. (3)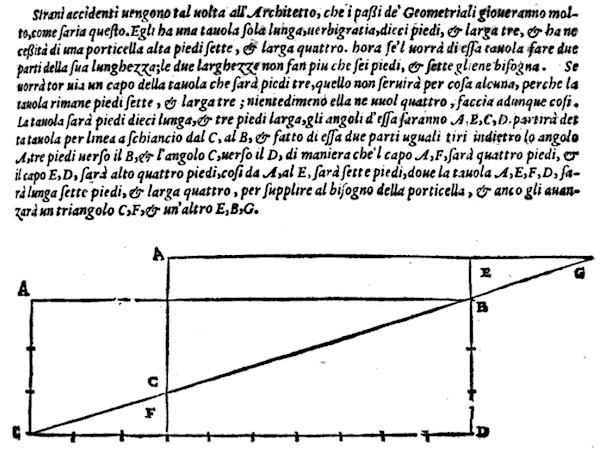

Sebastiano Serlio, Libro d’Architettura Primo, 1566, p. 16 (read it here)

He cites the case of a 3×10 rectangle which can be converted into a rectangle 4×7 and two triangles 1×3. Curiously, Serlio didn’t noticed that the sum of the three resulting areas (31 square feet) was greater than the original one (30 square feet):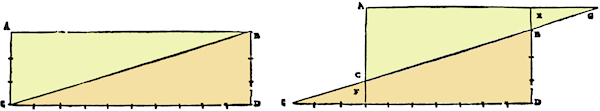

Edme Gilles Guyot’s explanation

In 1769 Edme Gilles Guyot (1706-1786) described the vanish area paradox in the second volume of his Nouvelles récréations physiques et mathématiques (read it here): a 10 × 3 square can be divided in four pieces which, rearranged, define a 5 × 4 and a 6 × 2 rectangle. (4)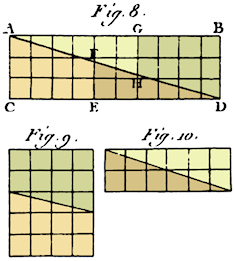

Edme Gilles Guyot, Nouvelles récréations physiques et mathématiques,
Vol.2, planche 6, 1799 edition (see here the planche 6)

Guyot suggested to draw a coin on each square and to use this paradox to tease alchemists and their claim of producing gold in mystical ways. Curious detail: the diagram given in the first edition of the book was wrong, and it was corrected in the second edition.

In the 1774 edition of Rational Recreations (5), a math puzzle book written by William Hooper (1742-1790) which partly plagiarized Guyot’s work (read it here), the error was repeated: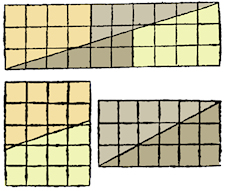

William Hooper, Rational Recreations, Volume 4, 1774, pp. 286-287. (read it here)

It was corrected in the 1782 edition of Hooper’s book (read it here).

The second rectangle was 3×6 instead of 2×6.

Mitsunobu Matsuyama’s Paradox

In 1979 Mitsunobu Matsuyama created “Paradox”, a simple vanishing area puzzle involving a playing card: the back of a playing card, when reversed, shows a King of Diamonds, augmented of a small additional square coming from nowhere.

It was published by Karl Fulves in the issue 18 of Chronicles (6)

Winston Freer Tile Puzzle

Winston Freer (1910-1981) created a more intricate version, used in this video by John Rogers to explain relativity theory: it is still more astonishing because it involves three different steps.

The structure of Freer’s puzzle is described in detail by Peter Tappan in “FuTILE Subtraction”. (7)

In the article, Peter Tappan describes an improved version of the vanishing area puzzle, which in 1994 won the I.B.M. Originality Contest.

Tofique Fatehi’s progression

In 1980 Tofique Fatehi created “The eleven holes puzzle”, an extended version of Winston Freer’s puzzle involving a progression of 11 vanishing squares!

It starts from here: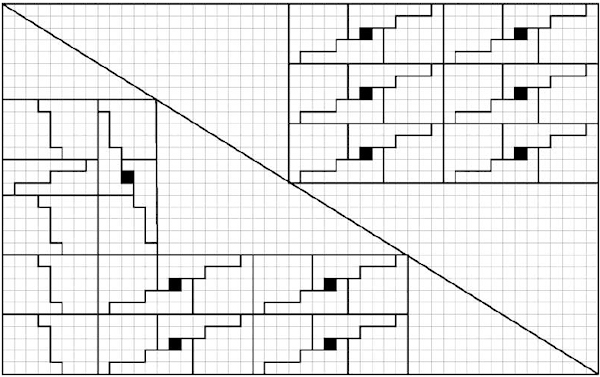

A detailed description of its structure can be downloaded from his website.

Robert Pages’s Business Card Paradox

Robert Page marketed a “Business Card Paradox”, a simplified version of Matsuyama’s Paradox in which the rectangular vanishing area was replaced with a personal business card.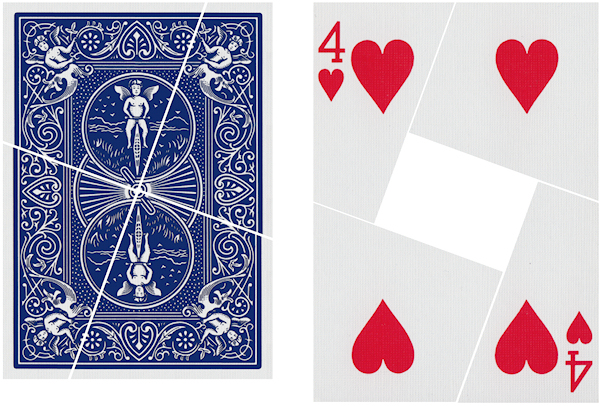

A tutorial is available to create a similar puzzle (see it on YouTube).

Gianni Sarcone’s TangraMagic

In 1998 Gianni Sarcone created TangraMagic, an extended Tangram involving a vanishing square, cut in foam. The 10 pieces (7 original and 3 additional) can be rearranged so that only 9 pieces fit the same area in a frame. (8)

His website offers a tutorial to create it.

Many thanks to Max Maven for his precious contributions to this article. (9) Gianni Sarcone also provided useful information about the topic.

_________________