MARIANO TOMATISWONDER INJECTORItalian writer and magician
|
 Magic & AlchemyThe 16th century computer and the book that kills Posted on friday 4 july 2014, 35 days before scifoo14 • Written by Mariano Tomatis Designing and recreating a wood-and-glass computer used in the 16th century? A funny activity with a certain amount of thrill, especially because the mathematical secret at its core could lead to death! It all started with the “Book of Soyga” (discover its story here), a 16th century treatise that included 18 leaves containing 36 tables of letters. Their structure has been shrouded in mystery for centuries, and — according to legend — revealing its secret would have brought to death in two years and a half. The alchemist and astrologer John Dee (1527-1608) invoked the archangel Uriel to solve the puzzle, but in vain. Four centuries later, the mathematician James A. Reeds defied the curse, discovering and publishing (1) in 2006 the method to compile all 36 tables of the “Book of Soyga”. How to fill in the “Book of Soyga” In order to create a page of the book, draw a square grid, consisting of 36 rows and 36 columns. From the upper left corner, write vertically a “magic word”, followed by itself upside down, then back upright, upside down, etc. until you have completed the column. Here’s how to fill it starting from ABRACADABRA: 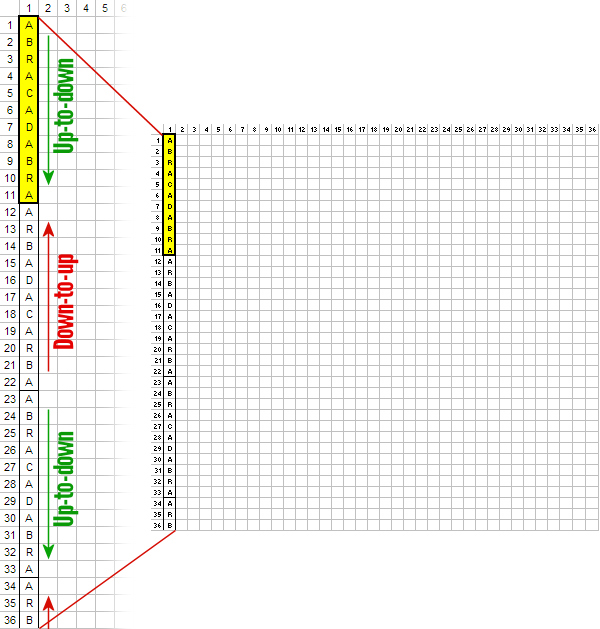 It is easy to fill in the first row. Reeds discovered that each letter is followed by another according to a simple rule: 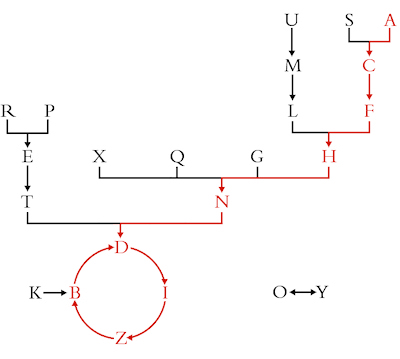 We start from the A which is in the top left square. The arrows in the diagram above show the obligatory path that leads from A to C, then to F, H, N and D, and then enters the closed circle I, Z, B and D again. Let’s write, on the first line, the path described by the rule: 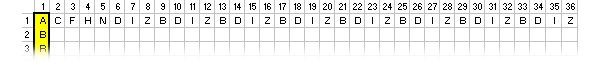 Now the procedure becomes more difficult. Each of the other squares should be filled on the basis of the letter above and the one on the left, according to a mathematical rule that Reeds summarized in this equation: 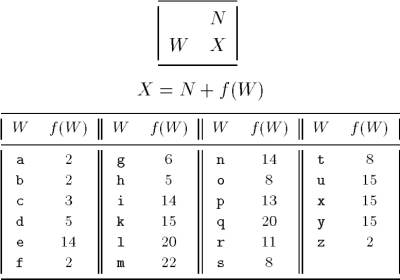 Complex enough, isn’t it? According to the equation, the unknown letter X is obtained starting from the (N)orthern letter and moving on in the alphabet a number of positions determined by the (W)estern letter. Each letter corresponds to a different shift, as indicated in the table above. (The procedure is better explained here.) Can you imagine the amount of work needed by the creator of the tables, who had to “calculate” the equation for more than 45,000 times? A modern computer can fill the table starting with ABRACADABRA in a fraction of a second (check it here), but a manual work would take months. What if they had used a computer in the 16th century? What if they had used a computer in the 16th century to compile the book? «Which kind of computer would have worked?», I asked myself. Inspired by the mnemonic wheels of Ramon Llull (1235-1316), already known at the time, and by the gift of a dear friend — David Metcalfe —, I designed a “Soyga Tables Compiler” consisting of two wheels, fixed on a wooden base: 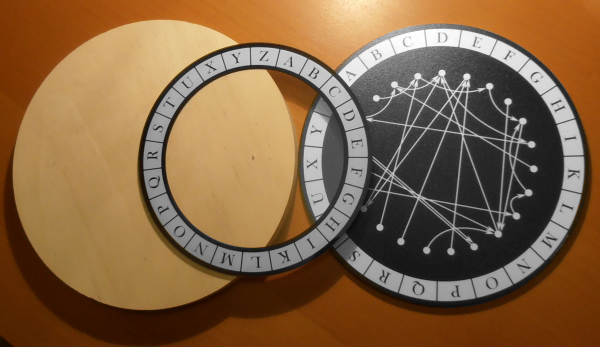 Both wheels show in the external crown the 23 letters of the alphabet appearing on the tables of the “Book of Soyga”. The right wheel shows, at the center, arrows leading from one letter to another. A second wheel is printed on glass and fixed in the center of the first. When spun, the arrows in the background remain fixed, and being transparent, different rotations produce different connections between letters. Here is the hypothetical 16th century computer:  Such an instrument would enable anyone to compile a table of the “Book of Soyga” ignoring the complex rules described by Reeds, because it incorporates them all in a single object easy to work with. (2) In order to compile the first line, it is enough to rotate the two wheels in a way that all the letters are aligned: the arrows thus obtained reproduce the graph above and allow you to easily see the path to follow — A leading to C, then to F, etc. 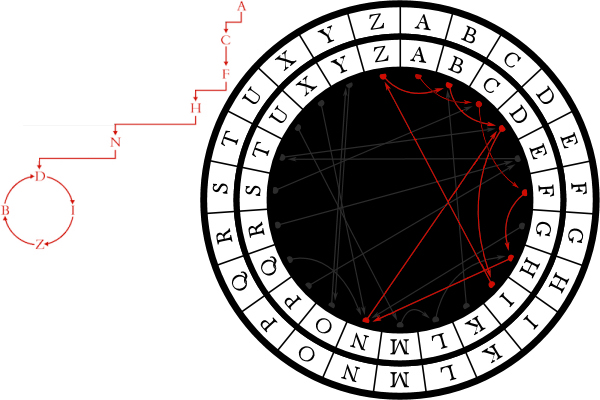 From the second row, a different rule applies, equally simple: in order to find out what to write in a square, the wheels must be rotated until the letter on the left (on the outer circle) and the letter above (in the inner ring) are aligned; now the arrow (red in the diagram) starting from the letter above leads to the letter to be written — in this case, to E: 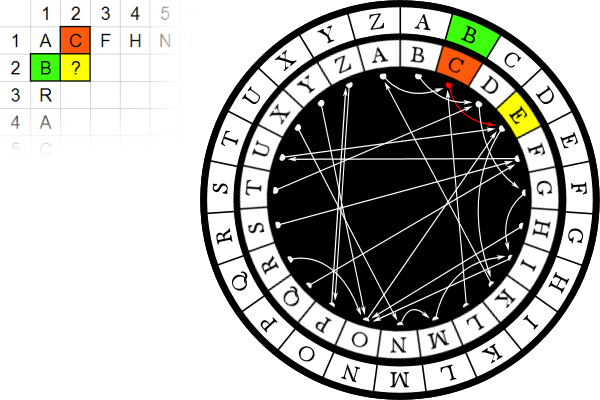 This procedure can be repeated for the entire table, square by square, and allows you to faithfully reconstruct the entire “Book of Soyga”. To better understand its use, click here: the table shown is based on the magic word ABRACADABRA, and clicking on any letter (from the second column on) you can see the interaction between the two wheels and — indicated by two red dots — the arrow that connects the letters involved in the encoding procedure: 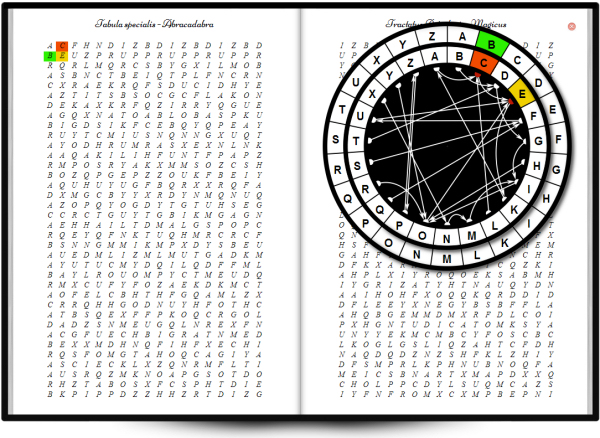 _________________ (1) Jim Reeds, “John Dee and the Magic Tables in the Book of Soyga” in Stephen Clucas (ed.), John Dee: Interdisciplinary Studies in English Renaissance Thought, International Archives of the History of Ideas, No. 193. Dordrecht, Netherlands: Springer, 2006, pages 177-204. The article can be downloaded from here. (2) I stress again that, to date, there is no description of a computer like that, which I designed in a totally hypothetical way, limiting myself to technologies and mathematical knowledges available at the time. You could be interested also in:
|
 Science Foo Camp (or “Sci Foo”) is an invitation-only gathering organized by Digital Science, O'Reilly Media, and Google, with support from Nature. The 9th edition of Sci Foo takes place on 8-10 August 2014 at the Googleplex in Mountain View, CA. Lord Martin Rees has defined it as “a sort of mini Woodstock of the Mind”. Participants include researchers, writers, educators, artists, policy makers, investors, and other thought leaders, all doing groundbreaking work in diverse areas of science and technology.
Science Foo Camp (or “Sci Foo”) is an invitation-only gathering organized by Digital Science, O'Reilly Media, and Google, with support from Nature. The 9th edition of Sci Foo takes place on 8-10 August 2014 at the Googleplex in Mountain View, CA. Lord Martin Rees has defined it as “a sort of mini Woodstock of the Mind”. Participants include researchers, writers, educators, artists, policy makers, investors, and other thought leaders, all doing groundbreaking work in diverse areas of science and technology.